Class 10 Maths Chapter 17
Mensuration
Important Questions

In ICSE Class 10 Mathematics, Chapter 17 focuses on "Mensuration." This chapter deals with the measurement of geometric figures, such as areas and volumes. It is an essential part of mathematics as it helps students understand and calculate the sizes and capacities of various shapes and objects.

Introduction

In Class 10 ICSE (Indian Certificate of Secondary Education) mathematics, the chapter on "Mensuration" is a fundamental topic that deals with the measurement of geometric figures, including calculating their areas and volumes. Mensuration is a practical and essential part of mathematics with numerous real-world applications. Mensuration is not just a mathematical concept; ICSE class 10 maths mensuration is a practical tool that helps us quantify and measure objects in our everyday lives. Whether you're designing a building, calculating the area of a land plot, or estimating the volume of a container, mensuration plays a vital role in solving real-world problems accurately. Here are some area and volume class 10 ICSE questions.

What is Mensuration?

Ans: Mensuration is the branch of mathematics that focuses on measuring the sizes, areas, and volumes of various geometric shapes and figures. ICSE class 10 maths mensuration provides us with the tools and formulas to quantify and understand the spatial properties of objects in both two and three dimensions.

Key Concepts and Objectives:

Area and Perimeter: Mensuration helps us calculate the area (space inside a shape) and perimeter (the boundary length) of 2D figures such as rectangles, squares, circles, and triangles.

Volume: It allows us to determine the volume (space occupied) of 3D figures like cubes, cuboids, cylinders, cones, and spheres.

Formulas: Mensuration provides us with specific formulas and methods for each type of shape, making it possible to compute measurements accurately.

Class 10 Mensuration Important Questions and Answers

Q1. Volume of a cone is 220 cm³. The volume of the cylinder having same radius and height as that of the given cone is:

Options

(a) 220 cm³
(b) 110 cm³
(c) 660 cm³
(d) 330 cm³

Ans. (C) 660 cm³

Explanation:
volume of cone = (1/3) πr²h = 220  cm³
Volume of cylinder = πr²h = 3×220  cm³ (∵ radius and height of cylinder are as same as that of cone)

Q2. If the circumference of a circle and the perimeter of a square are equal then:

Options

(a) area of the circle = area of the square
(b) area of the cricle > area of the square
(c) area of the circle < area of the square
(d) none of these

Ans. (b)
Explanation: Circumference of circle = 2πR
Perimeter of square = 4a
According to question 
2πR = 4a
⇒ πR = 2a
⇒ \(\frac{\pi R}{2}\)= a
\(Area of square = a^2 = \left(\frac{\pi R}{2}\right )^2 \) =\(\frac{\pi^2 R^2}{4}\)
\(\begin{bmatrix} \because \pi = 3.14 and 3.14 \lt 4 \\ \therefore \frac{3.14}{4}\lt 1\end{bmatrix}\)
π R²>\(\frac{\pi}{4}\)π R²
Area of circle > Area of square

Q3. Determine :
(i) The curved or lateral surface area of a cylindrical petrol storage tank that is 4·0 m in diameter and 4·4 m high.
(ii) How much steel was actually used, if \(\frac{1}{2}\) of the steel actually used was wasted in making the closed tank.

Explanation:
(i) Here,
r=\(\frac{4.0}{2}\) = 2m and h = 4⋅4 m
Curved surface area = 2πrh m²
=2×\(\frac{22}{7}\)×2×4⋅4 cm²
= 55.31 m²
(ii) Since \(\frac{1}{2}\) of the actual steel used was wasted, the area of the steel which has gone into the
tank =\(\begin{pmatrix}1- \frac{1}{12}\end{pmatrix}\)=\(\frac{11}{12}\)of x, where
x = total area of steel used.Steel used = (2πrh + 2πr²) m²
=(55⋅31+2×\(\frac{22}{7}\)×4) m²
= (55·31 + 25·14) m^2
‍= 80·45 m²
∴ \(\frac{11}{12}\)x =80.45
⇒                x = 87·76 m²
Hence, the actual area of the steel used = 87·76 m².

Q4. A circus tent is cylindrical to a height of 3 metres and conical above it. If its diameter is 105 m and the slant height of the conical portion is 53 m, calculate the length of the canvas which is 5 m wide to make the required tent.

Explanation:
Cylindrical area=2πrh
= 2 ×\(\frac{22}{7}\)×\(\frac{105}{2}\)×3 m²
=and conical area = πrl
=\(\frac{22}{7}\)×\(\frac{105}{2}\)× 53 m^2‍

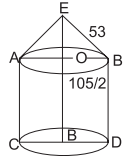
=2×\(\frac{22}{7}\)×\(\frac{105}{2}\)×3+\(\frac{22}{7}\)×\(\frac{22}{7}\)×\(\frac{105}{2}\)×53
=15×11(2×3+53)
=15×11×59
=165×59 m²
Length of canvas =\(\frac{165×59}{5}\)m
=33×59 m
=1947 m.

Q5. A circus tent consists of cylindrical base surmounted by a conical roof. The radius of the cylinder is 10 m. The height of the tent is 60 m and that of the cone is 20 m. Find the volume of the tent and the area of the canvas used for making it.

Explanation:
Height of the tent = Height of cone + Height of the cylinder

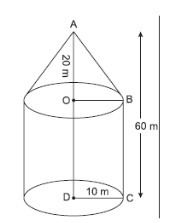
Given, height of tent = 60 m  
                           H = height of cone = 20 m
∴ Height of cylinder   
                             = h = 60 – 20 = 40 m
and Radius of cone = Radius of cylinder 
                             r = 10 m
∴ Volume of the tent = Volume of cylinder+ Volume of the cone
=πr²h + \(\frac{1}{3}\) πr²H
= πr² \(\begin{pmatrix}h+ \frac{H}{3}\end{pmatrix}\)
= π(10)² \(\begin{pmatrix}  40+ \frac{20}{3}\end{pmatrix}\)
=100 ×\(\frac{22}{7} \begin{pmatrix}\frac{140}{3}\end{pmatrix}\)
= 14666·6 m³
Slant height of the cone is
I=\(\sqrt{H^2+r^2}\)
=\(\sqrt{400+100}\)
=\(\sqrt{500}\)=\(\sqrt[10]{5}\)m
Since, curved surface area of cone
= πrl 
=\(\frac{22}{7}\)×10×10\(\sqrt{5}\)m²
and curved surface area of cylinder
= 2πrh
=2×\(\frac{22}{7}\)×10×40
∴ Total surface area of the canvas in making the tent
= C.S.A. of cylinder + C.S.A. of cone
= 2πrh + πrl
= πr (2h + l)
=\(\frac{22}{7}\)×10(2×40+10\(\sqrt{5}\))m²
=\(\frac{220}{7}\) (80+10 \(\sqrt{5}\))m²
Total Surface Area = 3217·04 m²

ICSE Class 10 Maths Chapter wise Important Questions

Conclusion

In conclusion, the chapter on ICSE class 10 maths mensuration is an integral part of the curriculum, offering students the essential knowledge and skills to measure and calculate areas and volumes of geometric shapes. These concepts extend far beyond the classroom, finding application in various real-world scenarios such as construction, design, and everyday problem-solving. Mastery of mensuration equips students with a valuable toolkit for understanding and quantifying the spatial attributes of objects, laying the foundation for more advanced mathematical concepts and practical life applications.
If you want to get better at this chapter and really understand it, check out oswal.io. They have lots of extra questions to help you practice and get a deeper grasp of the ideas. It's like having a treasure chest of knowledge to make you a math wizard!

Frequently Asked Questions

Q1 : What is mensuration in mathematics?

Ans: Mensuration in mathematics is the study of measuring and calculating the areas and volumes of geometric shapes and figures.

Q2: Why is mensuration important?

Ans:  Mensuration is crucial because it helps us quantify and understand the sizes of objects in real-world situations, including construction, design, and everyday problem-solving.

Q3 : What are some common 2D shapes studied in mensuration?

Ans: Common 2D shapes include rectangles, squares, circles, triangles, and parallelograms.

Q4 : What is the formula for finding the area of a rectangle?

Ans: The area of a rectangle is calculated by multiplying its length and width (A = length × width).

Q5 : How do you find the perimeter of a square?

Ans: The perimeter of a square is four times the length of one of its sides (P = 4 × side length).