logo
Log in
  Get Rs 200 off on Oswal Premium Plan for your board preperatoin! For Class 9th till 12th. Use Coupon Code BOARD200 at checkout.
logo
Log in
Start Free Trial
Real Number

Home / Boards / CBSE / Important Questions / Class 10 / Maths / Real Number

Class 10 Maths Chapter 1
Real Numbers Important Questions

exam preparation with oswal.io
Click here
Here are some important Class 10 Mathematics questions for Chapter 1, Real Numbers. These questions are designed to help students practice and score well in their CBSE Class 10 Mathematics Examination 2024-25. Practicing a variety of question types will help students clarify their doubts and prepare effectively for the exams. Solving these questions will not only build confidence but also enhance problem-solving skills.

Introduction

In Chapter 1 of Class 10 Mathematics, Real Numbers, you will review operations with real numbers from previous grades and learn about two significant properties of positive integers: Euclid’s Division Lemma and algorithm, as well as the fundamental theorem of arithmetic.

What are Real Numbers?

Real numbers encompass positive integers, negative integers, irrational numbers, and fractions, with the exception of complex numbers. This means that almost every number falls under the category of real numbers. Examples of real numbers include -1, ½, 1.75, √2, and others.
  • Real numbers consist of both rational and irrational numbers combined.
  • Every real number can be represented on the number line.
real number important questions and answers

Class 10 Real Numbers Important Questions and Answers

Q 1. The sum of exponents of prime factors in the prime-factorization of 196 is:
Options
(a) 3
(b) 4
(c) 5
(d) 2

Ans. (b)

Explanation:
Prime factorization is the method of representing a number as the multiplication of its prime factors. A number qualifies as prime if it possesses solely two factors , 1 and the number itself.Here's a breakdown of the sequential procedure for identifying the prime factors in the prime factorization of 196:
Prime factors of 196 = 2^2 \space × \space 7^2 \\ ‍So, sum of exponents = 2+2=4

Q 2. The total number of factors of a prime number is:
options

(a) 1
(b) 0
(c) 2
(d) 3

Ans. (c)

Explanation:
As we know that prime numbers are the numbers with two factors, 1 and the number itself.
∴ Total number of factors of a prime number will be 2.

Q 3. The LCM of two numbers is 14 times their HCF. The sum of LCM and HCF is 750. If one number is 250, then find the other number.

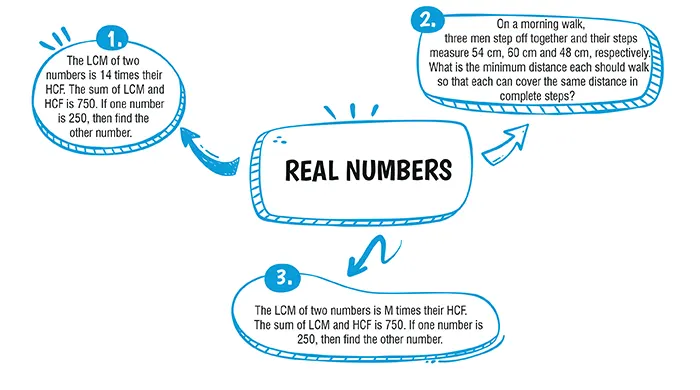
Explanation:
Let HCF be H.
Then      LCM = 14H
Now, sum of LCM and HCF is 750.     [Given]

∴     14H + H = 750
⇒    15H = 750
⇒ H \space = \space \frac{750}{15} \\ ⇒       H = 50
∴      LCM = 14H = 14 × 50 = 700
We know that,
Product of LCM and HCF = Product of two numbers.
Then,      700 × 50 = 250 × y
⇒ \space\space\space\space y \space = \space \frac{700 × 50}{250}\\ ⇒ Y \space = \space 140

Hence, the other number is 140.

Q 4. On a morning walk, three men step off together and their steps measure 54 cm, 60 cm and 48 cm, respectively. What is the minimum distance each should walk so that each can cover the same distance in complete steps?

Explanation:
The minimum distance that each should walk is the LCM of 54, 60 and 48.
Now, \space\space\space\space\space 54 = 2 × 3^3 \\ \space\space\space\space\space\space\space\space\space\space\space 60 = 2^2×3×5 \\ \space\space\space\space\space\space\space\space\space\space\space 48 = 2^4 × 3 \\ ∴ \space\space\space\space\space LCM (54, 60, 48) \space = \space 2^4 × 3^3 × 5    = 2160 \\ ∴ \space\space\space\space\space \text{Required distance  = 2160 cm} \\ \space\space\space\space\space\space\space\space\space = \space 21 m 60 cm

Q 5. The LCM of two numbers is M times their HCF. The sum of LCM and HCF is 750. If one number is 250, then find the other number.

Explanation:
Let HCF be ‘H’
Then LCM = 14H  sum of LCM and HCF is 750.
∴      14H + H  = 750
⇒      15H = 750
\Rightarrow \space\space\space\space \text{H} =\large\frac{750}{15}\\  ⇒      H = 50
∴      LCM = 14H
             = 14 × 50 = 700
We know
Product of LCM and HCF = Product of two numbers.
Let other number be y
Then,
700 × 50 = 250 × y
⇒  \text{y} = \large\frac{700 × 50}{250} ⇒ y = 140
Hence, the other number is 140.

Download PDF

CBSE Class 10 Maths Chapter wise Important Questions

making of global world class 10 important questions

Conclusion

If you are looking to further practice and enhance your understanding of the concepts discussed in the chapter, oswal.io provides a comprehensive set of questions for understanding the concept in a better way.

Frequently Asked Questions

Ans: Rational numbers can be expressed as a fraction of two integers, while irrational numbers cannot. Rational numbers have terminating or repeating decimal representations, whereas irrational numbers have non-repeating, non-terminating decimal representations.
Ans: Yes, real numbers can be negative. They include negative rational numbers (e.g., -1/2, -3/4) and negative irrational numbers (e.g., -√2, -π).
Ans: Yes, all integers (positive, negative, and zero) are real numbers. In fact, integers are a subset of rational numbers because they can be expressed as fractions with a denominator of 1.
Ans: The decimal representation of a real number is the representation of that number using decimal digits. It can be terminating (ends) or non-terminating (repeating or non-repeating).
Ans: No, not all square roots are irrational. The square root of perfect squares (like 4, 9, 16, etc.) is rational, while the square root of non-perfect squares (like 2, 3, 5, etc.) is irrational.
logo
Instagram Linkedin-in Facebook-f Youtube Twitter
logo
Instagram Linkedin-in Facebook-f Youtube Twitter

CBSE Important Questions Class 10

ICSE Important Questions Class 10

CBSE Important Questions Class 10

ICSE Important Questions Class 10

Contact Us

© Copyright 2025 oswal.io by
Oswal Publishers Simplifying Exams