logo
Log in
  Get Rs 200 off on Oswal Premium Plan this New Year! For Class 9th and 12th. Use Coupon Code NEWYEAR200 at checkout.
logo
Log in
Start Free Trial
Coordinate Geometry

Home / Boards / CBSE / Important Questions / Class 10 / Maths / Coordinate Geometry

Class 10 Math Chapter 7
Coordinate Geometry

exam preparation with oswal.io
Here are some essential Class 10 Mathematics questions for Chapter 7, Coordinate Geometry , carefully selected to assist students in preparing for the CBSE Class 10 Mathematics Examination in 2024-25. These thoughtfully curated questions cover various types of problems and aim to help students strengthen their problem-solving skills and gain a deeper understanding of the Coordinate Geometry chapter. By practicing this diverse range of questions, students can clarify any doubts they may have and improve their performance in this chapter.

Introduction

In this chapter, we will study concepts of coordinate geometry, graphs of linear equations, the distance formula, and the section formula for internal division.These concepts are essential in understanding the relationship between algebra and geometry.

What are Coordinate Geometry?

Coordinate Geometry is a branch of mathematics that deals with the study of geometrical figures using a coordinate system. It involves representing points, lines, and shapes on a plane using ordered pairs of numbers (x, y) called coordinates.
Coordinate geometry class 10 important questions and answers

Class 10 Coordinate Geometry Important Questions and Answers

Q1. In the second quadrant, for a point, the abscissais ........... and the ordinate is ........... .
Options
(a) positive, negative
(b) negative, positive
(c) negative, negative
(d) positive, positive

Ans.(b)

Explanation:
In the second quadrant, X-axis is negative and Y-axis is positive. Therefore, for a point the
abscissa is negative and the ordinate is positive.

Q2. What is the ratio in which the line joining the points (6, 4) and (1, – 7) is divided by the X-axis?
Options
(a) 5 : 4
(b) 4 : 7
(c) 7 : 4
(d) 4 : 5

Ans. (b)

Explanation:
Let, k : 1 be the ratio in which the X-axis divides the line joining (6,4) and (1,-7).
Let, P be the point on the X- axis that divides the line.
\text{Then} \space \space P(x,y)=\begin{pmatrix} \dfrac{k+6}{k+1},\dfrac{-7k+4}{k+1}\end{pmatrix} \\[4.5 bp] \text{As P(x,y) lies on X-axis } \space P(x,0)=\begin{pmatrix} \dfrac{k+6}{k+1},\dfrac{-7k+4}{k+1} \end{pmatrix} \\[4.5 bp] \text{[∵ x- coordinate will be (x,0)]} \\[4.5 bp] \Rightarrow \dfrac{-7k+4}{k+1}=0 \\[4.5 bp] ⇒   -7k+4 = 0 \\[4.5 bp] ⇒     7k=4 \\[4.5 bp] \Rightarrow k= \dfrac{4}{7} \\[4.5 bp] \text{So, the required ratio be} \space \space k : 1 =\dfrac{4}{7}:1= 4 : 7

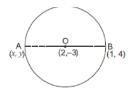
Q3. Find the coordinates of point A, where AB is the diameter of a circle whose center is (2, – 3) and B is the point (1,4).

Explanation:
Let the coordinates of point A be (x,y) and point O (2,-3) be the center,
then,
\text{By mid-point formula,}\space\space\space\space\space\space\space\space \dfrac{x+1}{2}=\text{2 and }\dfrac{y+4}{2}=-3 \\[4.5 bp] or      x = 4 - 1 and y =- 6 - 4
\Rightarrow \space\space\space\space\space\space\space\space \text{x = 3  and y = - 10} \\[4.5 bp]

questions on triangles for class 10
\therefore \space\space\space\space\space\space \text{The coordinates of point A are (3,- 10)}
Q4. The x-coordinate of a point P is twice its y-coordinate. If P is equidistant from Q(2, – 5) and R(– 3, 6), find the coordinates of P.

Explanation:
Let the coordinates of point P be (2y, y). Since , P is equidistant from Q and R.
\therefore \space\space\space\space\space\space\space\space PQ = PR \\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space \sqrt{(2y-2)^2+(y+5)^2}=\sqrt{(2y+3)^2 + (y-6)^2}\\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space (2y-2)^2 + (y + 5)^2= (2y + 3)^2 + (y - 6)^2 \\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space 4y^2 + 4 - 8y + y^2 + 25 + 10y = 4y^2 + 9 + 12y  + y^2 + 36 - 12y \\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space 2y + 29 = 45 \\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space 2y = 45 - 29 \\[4.5 bp] \Rightarrow \space\space\space\space\space\space\space\space y=\dfrac{16}{2} = 8

Hence, the coordinates of point P are (16,8).

Q5. Determine the ratio in which the line x – y – 2 = 0 divides the line segment joining the points A(3, – 1) and B(8, 9).

Explanation:
Given,      A = (3, – 1), B = (8, 9) and x – y – 2 = 0

Let the line x – y – 2 = 0 intersect the line segment AB at R in the ratio p : 1.

Now,   Rx = \dfrac{1(3)+p(8)}{p+1} \space \space and \space \space R_y =\dfrac{1(-1)+p(9)}{p+1} \\[4.5 bp] \Rightarrow \space\space R_x =\dfrac{3+8p}{p+1} \space\space and \space\space R_y =\dfrac{-1+9p}{p+1}\\[4.5 bp] \text{Thus,} \space \space R = \begin{pmatrix} \dfrac{3+8p}{p+1},\dfrac{9p-1}{p+1} \end{pmatrix} \\[4.5 bp]

Now substituting the value of R in the given equation,

\text{we get} \\[4.5 bp] \dfrac{3+8p}{p+1}-\dfrac{9p-1}{p+1}-2=0 \\[4.5 bp] \Rightarrow\space\space\space\space 3 + 8p – 9p + 1 – 2(p + 1) = 0 \\[4.5 bp] \Rightarrow\space\space\space\space 4 – p – 2p – 2 = 0 \\[4.5 bp] \Rightarrow\space\space\space\space 3p = 2 \\[4.5 bp] \Rightarrow\space\space\space\space P=\dfrac{2}{3}\\[4.5 bp] \text{Thus, the ratio is} \space \dfrac{2}{3} : 1 = 2 : 3.
Download PDF

CBSE Class 10 Maths Chapter wise Important Questions

making of global world class 10 important questions

Conclusion

To improve your understanding of the chapter’s concepts, oswal.io offers a wide range of practice questions. Their extensive question bank is designed to help you reinforce your knowledge and enhance your problem-solving skills. By using these resources, you can strengthen your grasp of the topics and become better at solving various types of problems.

Frequently Asked Questions

Ans: Coordinate Geometry is a branch of mathematics that involves the study of geometric figures using coordinate systems. It relates algebraic equations to geometric shapes and positions them on a coordinate plane.
Ans: The coordinates of the origin are (0, 0). It is the point where the x-axis and y-axis intersect.
Ans: The distance between two points (x_1, y_1) and (x_2, y_2) on the coordinate plane can be calculated using the Distance Formula: Distance = √[(x₂ – x₁)² + (y₂ – y₁)²]
Ans: The Section Formula for internal division gives the coordinates (x, y) of a point P that divides a line segment joining two points A(x₁, y₁) and B(x₂, y₂) in a given ratio ‘m:n’ internally as: x = [(mx₂ + nx₁)/(m + n)], y = [(my₂ + ny₁)/(m + n)]
Ans: The x-axis and y-axis are essential components of the coordinate plane. The x-axis represents the horizontal axis, and the y-axis represents the vertical axis. They intersect at the origin (0, 0). The coordinates of any point on the plane are determined by its position along these two axes.
logo
Instagram Linkedin-in Facebook-f Youtube Twitter
logo
Instagram Linkedin-in Facebook-f Youtube Twitter

CBSE Important Questions Class 10

ICSE Important Questions Class 10

CBSE Important Questions Class 10

ICSE Important Questions Class 10

Contact Us

© Copyright 2024 oswal.io by OSWAL PUBLISHERS Simplifying Exams